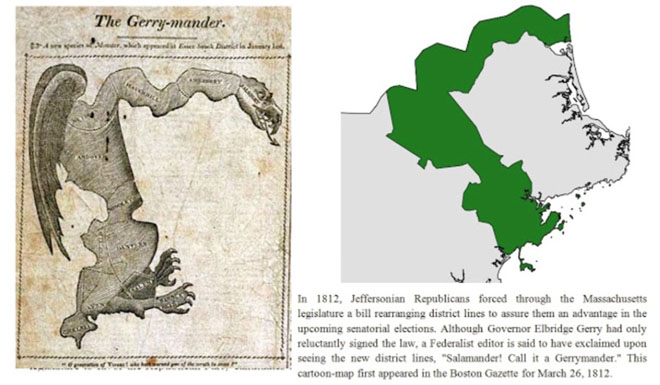
Left: Cartoon by Elkanah Tisdale from the March 26, 1812 edition of the Federalist-leaning paper The Boston Gazette showing the Massachusetts district newly created to favor the Jeffersonian Republicans in upcoming elections. Right: the wing and clawless version of the map.  Thinking the actual district looked like a salamander, an editor at the paper declared the creature a Gerry-mander, after Eldridge Gerry, the Governor who signed the redistricting bill into law. That it is drawn as a dragon is, I believe, another level of editorializing, or just a miscommunication between editor and illustrator, rather than zoological confusion. Source: Article the First by Stan Klos

In 2016, Moon Duchin, along with Mira Bernstein, Ari Nieh, Justin Solomon, and Michael Sarahan, created the METRIC GEOMETRY AND GERRYMANDERING GROUP.  MGGG

Anna Nowogrodzki, writing for Tufts Now, the newsletter of Tufts University where Drs. Duchin and Bernstein are on the faculty, introduces the group:

“The esoteric world of pure math doesn’t usually play much of a role in promoting fairness in the U.S. political system, but Tufts mathematicians Moon Duchin and Mira Bernstein believe that needs to change. It is math, they say, that could help overcome gerrymandering—the practice of drawing legislative districts that favor one party, class or race.”  Source: Tufts Now 19 July 2018.

Gerrymandering in theory (source: Siva Narayan)

Gerrymandering in practice (source: District 7, PA: